Mathematics Support

Representing Situations of the Form PX+Q=R and P(X+Q)=R

In this unit, your student will be representing situations with diagrams and equations. There are two main categories of situations with associated diagrams and equations.

Here is an example of the first type: A standard deck of playing cards has four suits. In each suit, there are 3 face cards and x other cards. There are 52 total cards in the deck. A diagram we might use to represent this situation is:

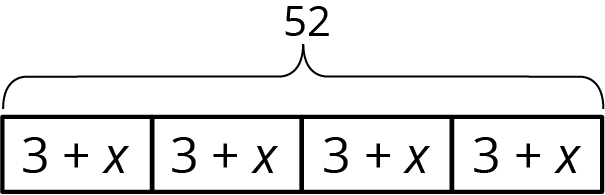
and its associated equation could be 52=4(3+x). There are 4 groups of cards, each group contains x+3 cards, and there are 52 cards in all.

Here is an example of the second type: A chef makes 52 pints of spaghetti sauce. She reserves 3 pints to take home to her family, and divides the remaining sauce equally into 4 containers. A diagram we might use to represent this situation is:

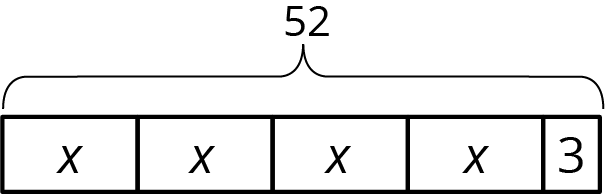
and its associated equation could be 52=4x+3. From the 52 pints of sauce, 3 were set aside, and each of 4 containers holds x pints of sauce.

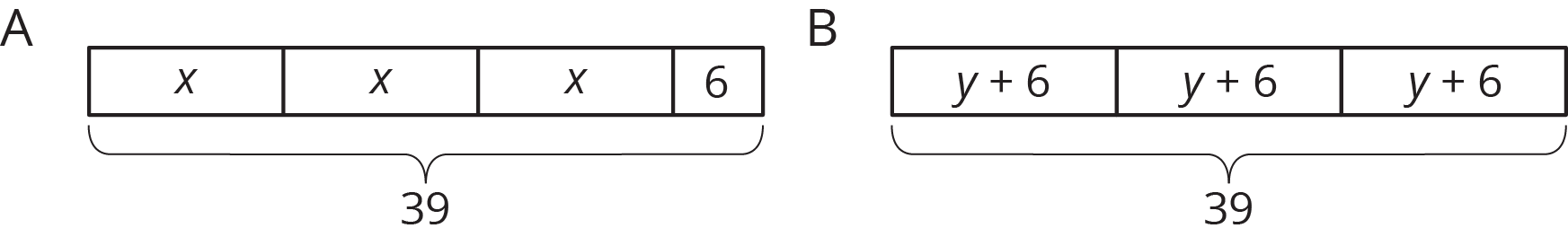
Here is a task to try with your student:

1. Draw a diagram to represent the equation 3x+6=39
2. Draw a diagram to represent the equation 39=3(y+6)
3. Decide which story goes with which equation-diagram pair:
   • Three friends went cherry picking and each picked the same amount of cherries, in pounds. Before they left the cherry farm, someone gave them an additional 6 pounds of cherries. Altogether, they had 39 pounds of cherries.
   • One of the friends made three cherry tarts. She put the same number of cherries in each tart, and then added 6 more cherries to each tart. Altogether, the three tarts contained 39 cherries.
    

Solution:

Diagram A represents 3x+6=39 and the story about cherry picking. Diagram B represents 3(y+6)=39 and the story about making cherry tarts. 

Solving Equations of the Form PX+Q=R and P(X+Q)=R and Problems that Lead to Those Questions

Your student is studying efficient methods to solve equations and working to understand why these methods work. Sometimes to solve an equation, we can just think of a number that would make the equation true. For example, the solution to 12−c=10 is 2, because we know that 12−2=10. For more complicated equations that may include decimals, fractions, and negative numbers, the solution may not be so obvious.

An important method for solving equations is doing the same thing to each side. For example, let's show how we might solve -4(x−1)=20 by doing the same thing to each side.

-4(x−1)-14⋅-4(x−1)x−1x−1+1x=24=-14⋅24=-6=-6+1=-5multiply each side by -14 add 1 to each side

Another helpful tool for solving equations is to apply the distributive property. In the example above, instead of multiplying each side by -14, you could apply the distributive property to -4(x−1) and replace it with -4x+4. Your solution would look like this:

-4(x−1)-4x+4-4x+4−4-4x-4x÷-4x=24=24=24−4=20=20÷-4=-5apply the distributive propertysubtract 4 from each sidedivide each side by -4

Here is a task to try with your student:

Elena picks a number, adds 45 to it, and then multiplies by 12. The result is 29. Elena says that you can find her number by solving the equation 29=12(x+45).

Find Elena’s number. Describe the steps you used.

Solution:

Elena’s number was 13. There are many different ways to solve her equation. Here is one example:

292⋅295858−4513=12(x+45)=2⋅12(x+45)=x+45=x+45−45=xmultiply each side by 2subtract 45 from each side

Inequalities

This week your student will be working with inequalities (expressions with >or < instead of =). We use inequalities to describe a range of numbers. For example, in many places you need to be at least 16 years old to be allowed to drive. We can represent this situation with the inequality a≥16. We can show all the solutions to this inequality on the number line.

Here is a task to try with your student:

Noah already has $10.50, and he earns $3 each time he runs an errand for his neighbor. Noah wants to know how many errands he needs to run to have at least $30, so he writes this inequality:

We can test this inequality for different values of e. For example, 4 errands is not enough for Noah to reach his goal, because 3⋅4+10.50=22.5, and $22.50 is less than $30.

1. Will Noah reach his goal if he runs:
   1. 8 errands?
   2. 9 errands?
    
2. What value of e makes the equation 3e+10.50=30 true?
3. What does this tell you about all the solutions to the inequality 3e+10.50≥30?
4. What does this mean for Noah’s situation?

Solutions 

1.  
   1. Yes, if Noah runs 8 errands, he will have 3⋅8+10.50, or $34.50.
   2. Yes, since 9 is more than 8, and 8 errands was enough, so 9 will also be enough.
    
2. The equation is true when e=6.5. We can rewrite the equation as 3e=30−10.50, or 3e=19.50. Then we can rewrite this as e=19.50÷3, or e=6.5.
3. This means that when e≥6.5 then Noah’s inequality is true.
4. Noah can’t really run 6.5 errands, but he could run 7 or more errands, and then he would have more than $30.

Writing Equivalent Expressions

This week your student will be working with equivalent expressions (expressions that are always equal, for any value of the variable). For example, 2x+7+4x and 6x+10−3 are equivalent expressions. We can see that these expressions are equal when we try different values for x.

We can also use properties of operations to see why these expressions have to be equivalent—they are each equivalent to the expression 6x+7.

Here is a task to try with your student:

Match each expression with an equivalent expression from the list below. One expression in the list will be left over. 

1. 5x+8−2x+1
2. 6(4x−3)
3. (5x+8)−(2x+1)
4. -12x+9

List:

• 3x+7
• 3x+9
• -3(4x−3)
• 24x+3
• 24x−18

Solution

1. 3x+9 is equivalent to 5x+8−2x+1, because 5x+-2x=3x and 8+1=9.
2. 24x−18 is equivalent to 6(4x−3), because 6⋅4x=24x and 6⋅-3=-18.
3. 3x+7 is equivalent to (5x+8)−(2x+1), because 5x−2x=3x and 8−1=7.
4. -3(4x−3) is equivalent to -12x+9, because -3⋅4x=-12x and -3⋅-3=9.